Difference in Means Confidence Interval Formula Explained
Input μ0 𝜎1 X 1-0992005 to a Z-score calculator and get Z 2576. For estimating the mean there are two types of confidence intervals that can be used.
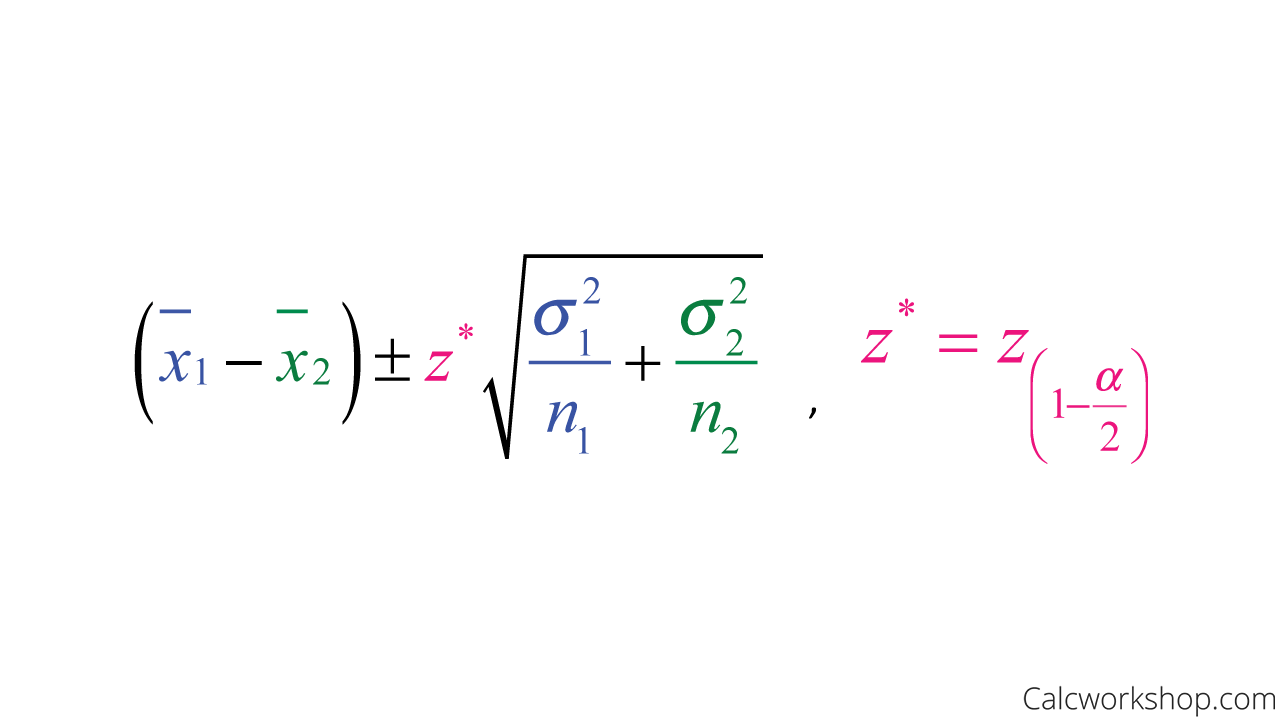
Confidence Intervals For Difference In Means 7 Examples
Since the formula has sqrtn in the denominator the larger the number of samples you have smaller will be the margin of error and thereby the smaller the confidence interval will be which also means more precise the estimate will be.
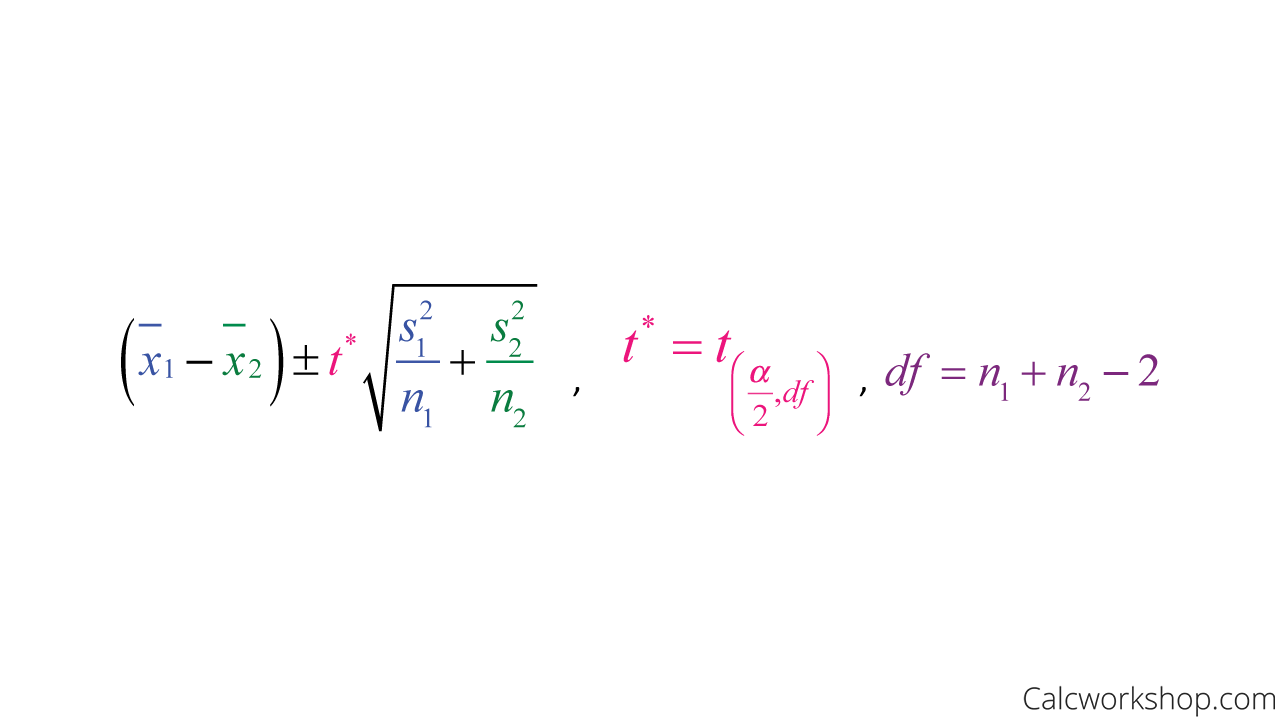
. To generate a confidence interval for a discrepancy in population means use the formula below. Here Xbar is the sample mean mean of the 1000 heights sample you took. The formulas are shown in Table 65 and are identical to those we presented for estimating the mean of a single sample except here we focus on difference scores.
Confidence level 1 a. The simple formula. The confidence level is a figure that represents the certainty a statistician has that if they repeat the same study or sample theyll receive the same results.
The formula to calculate the confidence interval is. Computing the Confidence Intervals for μ d. Ci_func.
Z is the no of standard deviations away from the sample mean 196 for 95 2576 for 99 level of confidence you want. Confidence Interval 330 233 05 100 to 330 233 05 100 Therefore the confidence interval at. Z Confidence coefficient.
If we were to repeatedly make new estimates using exactly the same procedure by drawing a new sample conducting new interviews calculating new estimates and new confidence intervals the confidence intervals would contain the average of all the. Confidence Interval for a Difference in Means. Confidence interval x1x2 - t sp2n1 sp2n2 where.
Confidence Interval x t α2 Sn Where n Number of terms. For example if you are estimating a 95 confidence interval around the mean proportion of female babies born every year based on a random sample of babies you might find an upper bound of 056 and a lower bound of. A confidence interval for a difference between means is a range of values that is likely to contain the true difference between two population means with a certain level of confidence.
The appropriate formula for the confidence interval for the mean difference depends on the sample size. The confidence interval consists of the upper and lower bounds of the estimate you expect to find at a given level of confidence. It is important to note that all values in the confidence interval are equally likely estimates of the true value of μ 1-μ 2.
N is the size of the sample. Sample 1 mean sample 2 mean. X Sample Mean.
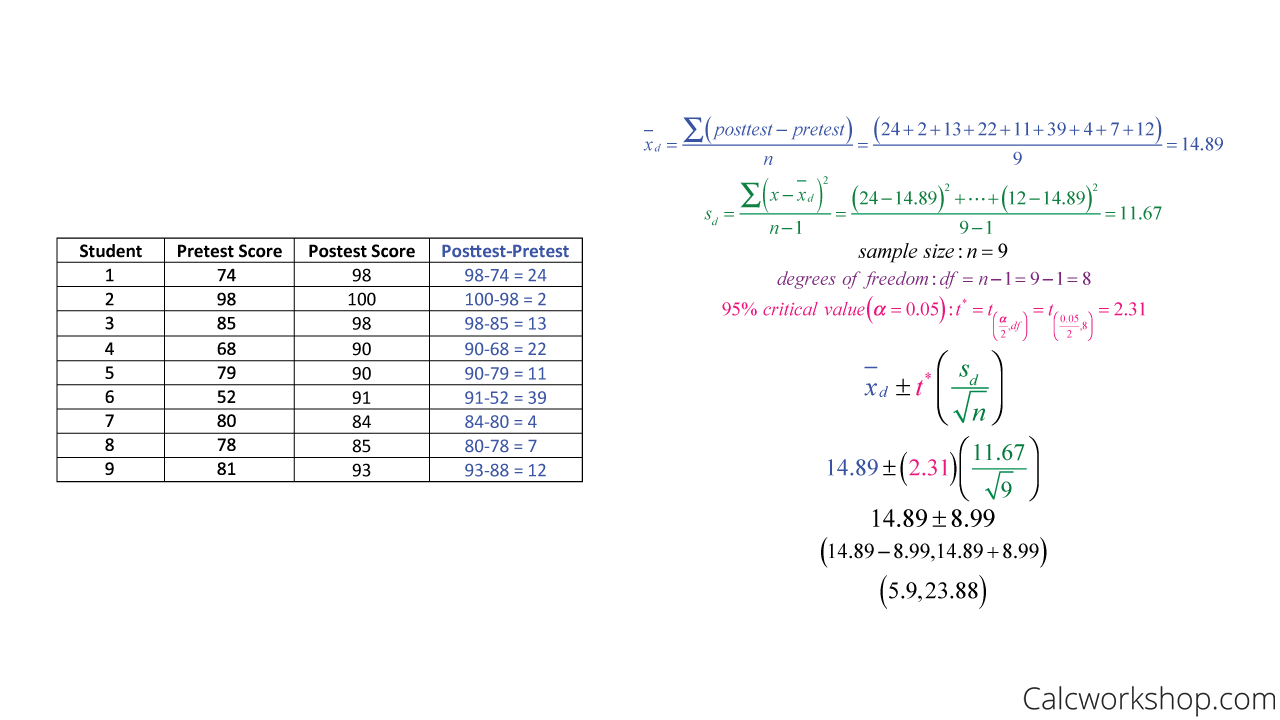
An experiment ws designed to estimate the mean difference in weight gain for pigs fed ration A as compared with those fed ration B. Technical Details There are two formulas for calculating a confidence interval for the difference between two population means. To find a confidence interval for the average difference between these two populations we compute textStandard Error for Difference sqrt0103204652 approx 0476 If we think about all possible ways to draw a sample of 60 boys under 10 and 600 men from 30-39 then the differences wed see between sample means would approximately follow the normal curve.
Though they function in statistics together theyre slightly different. σ Standard deviation. In this tutorial we will discuss how to determine confidence interval for the difference in means for dependent samples.
Confidence interval x1 x2 - t s p2 n 1 s p2 n 2 where. Confidence Interval 330 196 05 100 to 330 196 05 100 Therefore the Confidence Interval at a 95 confidence level is 320 to 340. If there is no difference between the population means then the difference will be zero ie μ 1-μ 2 0.
Eight pairs of pigs were used. Follow this question to receive notifications. The value after the symbol is known as the margin of error.
Sample 1 mean sample 2 mean. The confidence intervals for the difference in means provide a range of likely values for μ 1-μ 2. Confidence Interval for paired t-test.
Get Z-value from the 99 of the Confidence Level by assume μ0 𝜎1. Thus the formula to find CI is. Controls the probability that the distance from the difference in means to the confidence limits will be less than or equal to the value specified.
N sample space. Confidence interval x1x2-tsp2n1sp2n2 where. σ Standard Deviation.
The t-critical value based on the confidence level and n1n2-2 degrees of freedom. Z α2 Value corresponding to α2 in z table. A confidence interval is a way of using a sample to estimate an unknown population value.
As this interval does not contain zero I can be confident that I have used a method that that will produce significantly different or unequal population means 95 of the time. Your desired confidence level is usually one minus the alpha a value you used in your statistical test. The confidence interval for mean difference in life satisfaction for the two groups is -35346-8570.
α Confidence level. If n 30. The 90 in the confidence interval listed above represents a level of certainty about our estimate.
The confidence interval is based on the mean and standard deviation. Confidence Interval x z α2 σn If n. Sample 1 mean sample 2 mean.
S is the standard deviation in the sample. So if you use an alpha value of p 005 for statistical significance then your confidence level would be 1 005 095 or 95. The t-critical value based on the confidence level and n1n2-2 degrees of freedom.
We use the following formula to calculate a confidence interval for a difference between two means. In the following lesson we will look at how to. X Zα2 σ n Where.
The first difference between confidence levels and confidence intervals is their definitions. T α2 Value corresponding to α2 in t table.
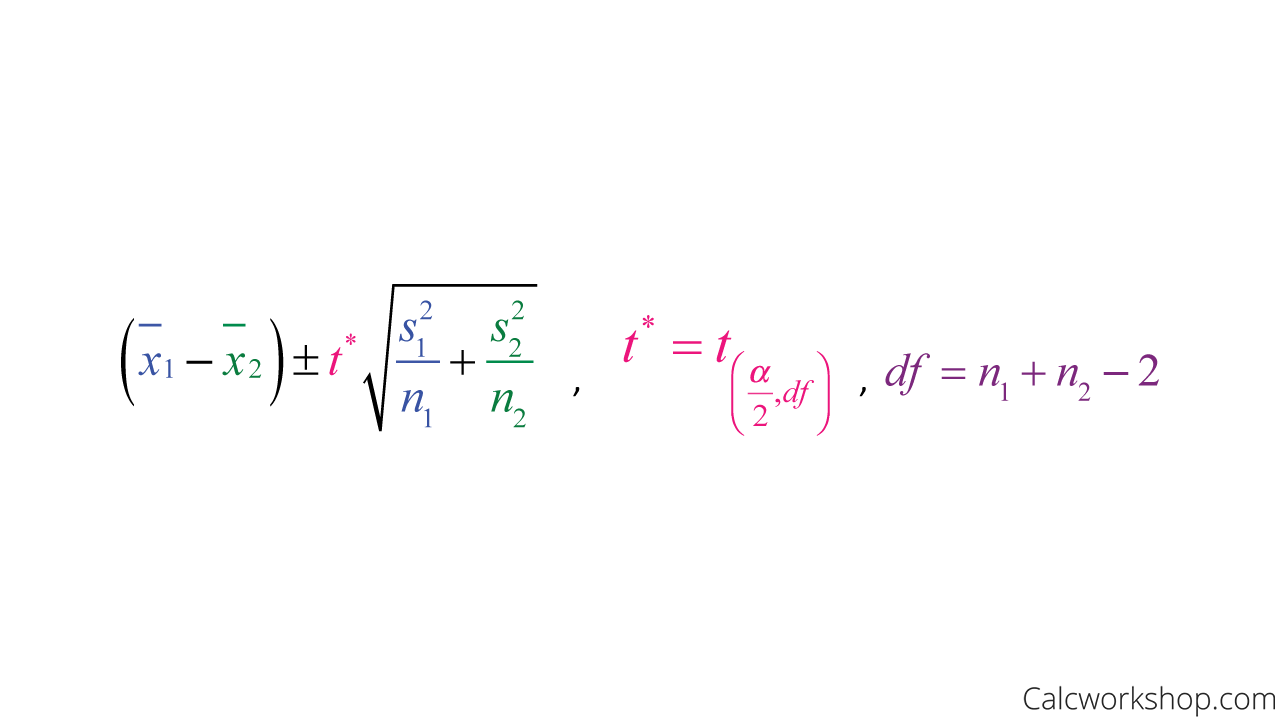
Confidence Intervals For Difference In Means 7 Examples
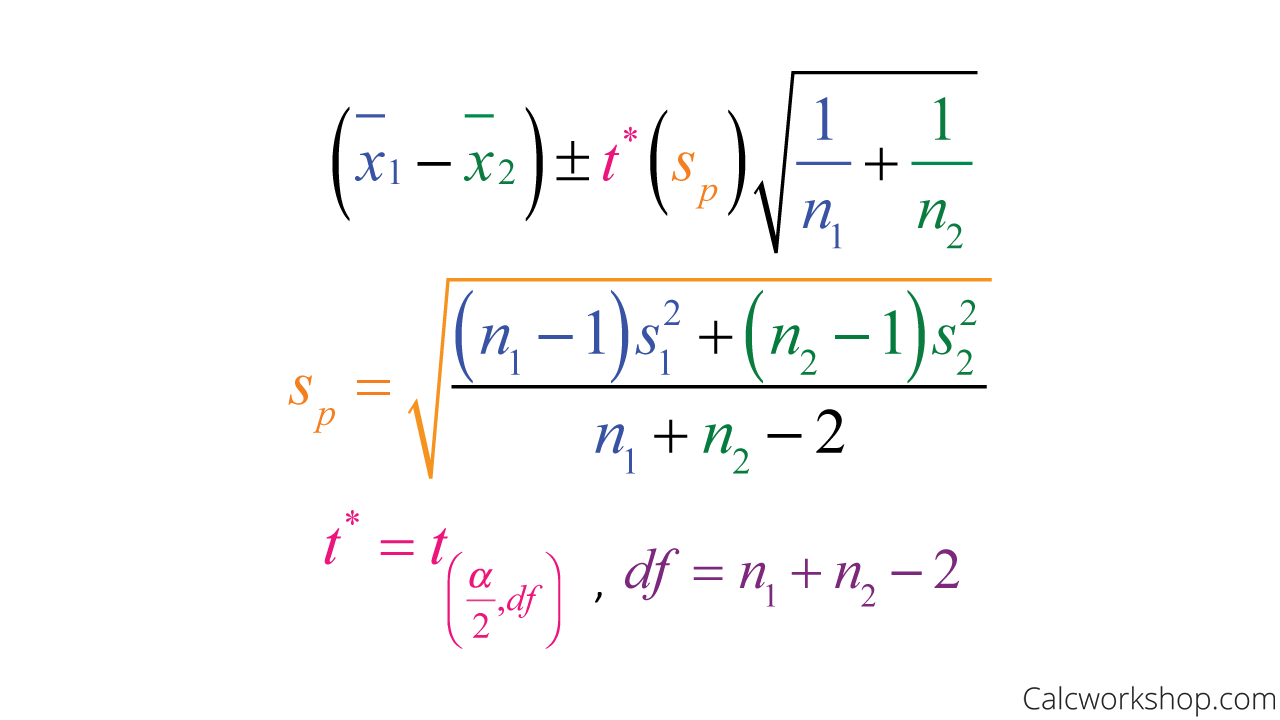
Comments
Post a Comment